Sturm Liouville Form
Sturm Liouville Form - If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. Where is a constant and is a known function called either the density or weighting function. The boundary conditions (2) and (3) are called separated boundary. P(x)y (x)+p(x)α(x)y (x)+p(x)β(x)y(x)+ λp(x)τ(x)y(x) =0. However, we will not prove them all here. If the interval $ ( a, b) $ is infinite or if $ q ( x) $ is not summable. We just multiply by e − x : Web essentially any second order linear equation of the form a (x)y''+b (x)y'+c (x)y+\lambda d (x)y=0 can be written as \eqref {eq:6} after multiplying by a proper factor. Web so let us assume an equation of that form. Web solution the characteristic equation of equation 13.2.2 is r2 + 3r + 2 + λ = 0, with zeros r1 = − 3 + √1 − 4λ 2 and r2 = − 3 − √1 − 4λ 2.
Web it is customary to distinguish between regular and singular problems. Put the following equation into the form \eqref {eq:6}: E − x x y ″ + e − x ( 1 − x) y ′ ⏟ = ( x e − x y ′) ′ + λ e − x y = 0, and then we get ( x e − x y ′) ′ + λ e − x y = 0. The boundary conditions (2) and (3) are called separated boundary. We apply the boundary conditions a1y(a) + a2y ′ (a) = 0, b1y(b) + b2y ′ (b) = 0, If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. There are a number of things covered including: P, p′, q and r are continuous on [a,b]; Share cite follow answered may 17, 2019 at 23:12 wang All the eigenvalue are real
Web the general solution of this ode is p v(x) =ccos( x) +dsin( x): If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. The functions p(x), p′(x), q(x) and σ(x) are assumed to be continuous on (a, b) and p(x) >. Basic asymptotics, properties of the spectrum, interlacing of zeros, transformation arguments. Put the following equation into the form \eqref {eq:6}: Web solution the characteristic equation of equation 13.2.2 is r2 + 3r + 2 + λ = 0, with zeros r1 = − 3 + √1 − 4λ 2 and r2 = − 3 − √1 − 4λ 2. Web it is customary to distinguish between regular and singular problems. P and r are positive on [a,b]. P(x)y (x)+p(x)α(x)y (x)+p(x)β(x)y(x)+ λp(x)τ(x)y(x) =0. P, p′, q and r are continuous on [a,b];
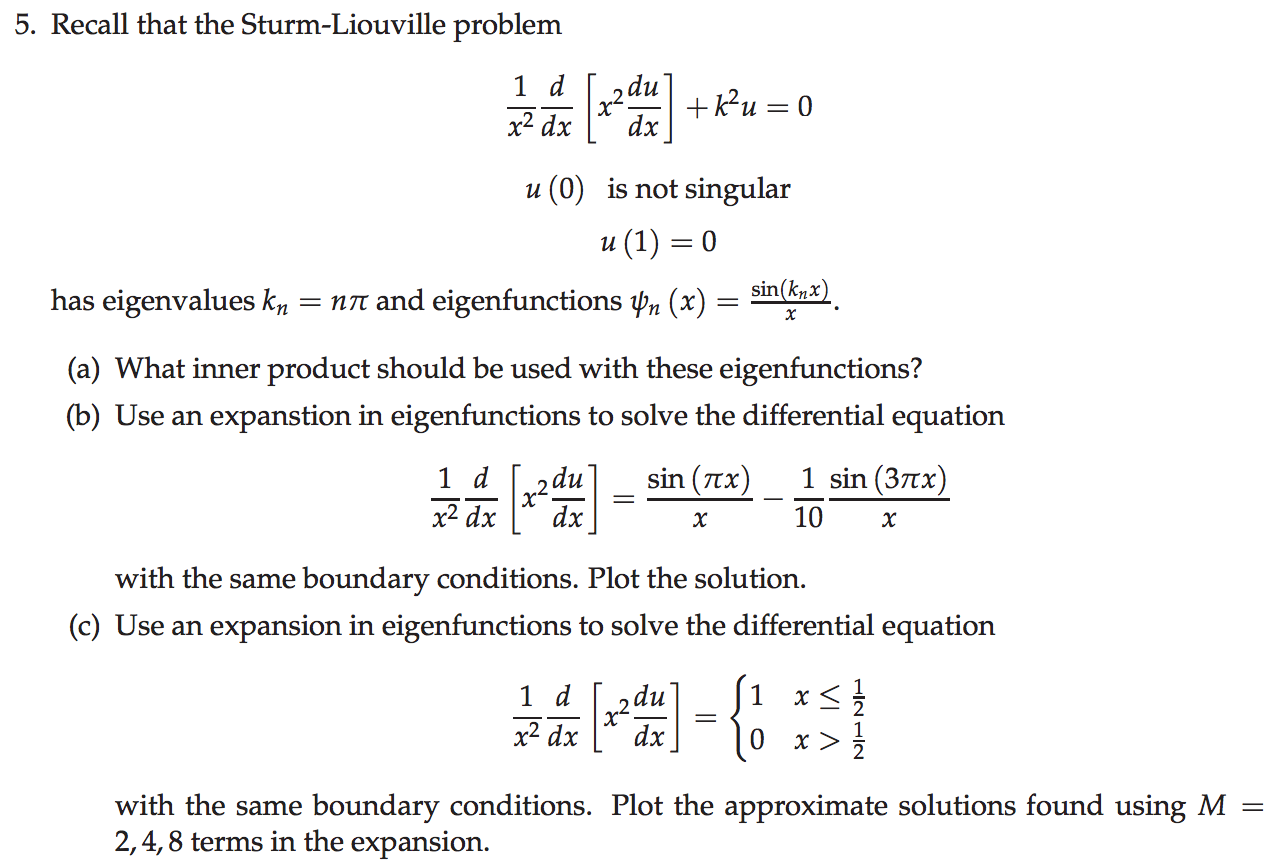
5. Recall that the SturmLiouville problem has
E − x x y ″ + e − x ( 1 − x) y ′ ⏟ = ( x e − x y ′) ′ + λ e − x y = 0, and then we get ( x e − x y ′) ′ + λ e − x y = 0. If the interval $ ( a,.
calculus Problem in expressing a Bessel equation as a Sturm Liouville
If the interval $ ( a, b) $ is infinite or if $ q ( x) $ is not summable. We will merely list some of the important facts and focus on a few of the properties. Put the following equation into the form \eqref {eq:6}: Web so let us assume an equation of that form. Where is a constant.
Putting an Equation in Sturm Liouville Form YouTube
If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. We will merely list some of the important facts and focus on a few of the properties. The most important boundary conditions of this form are y ( a).
MM77 SturmLiouville Legendre/ Hermite/ Laguerre YouTube
Where α, β, γ, and δ, are constants. P, p′, q and r are continuous on [a,b]; Share cite follow answered may 17, 2019 at 23:12 wang There are a number of things covered including: Web the general solution of this ode is p v(x) =ccos( x) +dsin( x):
20+ SturmLiouville Form Calculator NadiahLeeha
Where α, β, γ, and δ, are constants. If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. The boundary conditions require that P, p′, q and r are continuous on [a,b]; All the eigenvalue are real
SturmLiouville Theory YouTube
The most important boundary conditions of this form are y ( a) = y ( b) and y ′ ( a) = y. Where is a constant and is a known function called either the density or weighting function. E − x x y ″ + e − x ( 1 − x) y ′ ⏟ = ( x e.
Sturm Liouville Form YouTube
The solutions (with appropriate boundary conditions) of are called eigenvalues and the corresponding eigenfunctions. Web solution the characteristic equation of equation 13.2.2 is r2 + 3r + 2 + λ = 0, with zeros r1 = − 3 + √1 − 4λ 2 and r2 = − 3 − √1 − 4λ 2. P and r are positive on [a,b]..
20+ SturmLiouville Form Calculator SteffanShaelyn
If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. Web 3 answers sorted by: Basic asymptotics, properties of the spectrum, interlacing of zeros, transformation arguments. We just multiply by e − x : Web so let us assume.
SturmLiouville Theory Explained YouTube
We can then multiply both sides of the equation with p, and find. The functions p(x), p′(x), q(x) and σ(x) are assumed to be continuous on (a, b) and p(x) >. Web solution the characteristic equation of equation 13.2.2 is r2 + 3r + 2 + λ = 0, with zeros r1 = − 3 + √1 − 4λ 2.
Sturm Liouville Differential Equation YouTube
Put the following equation into the form \eqref {eq:6}: Basic asymptotics, properties of the spectrum, interlacing of zeros, transformation arguments. Web essentially any second order linear equation of the form a (x)y''+b (x)y'+c (x)y+\lambda d (x)y=0 can be written as \eqref {eq:6} after multiplying by a proper factor. If the interval $ ( a, b) $ is infinite or if.
Web Essentially Any Second Order Linear Equation Of The Form A (X)Y''+B (X)Y'+C (X)Y+\Lambda D (X)Y=0 Can Be Written As \Eqref {Eq:6} After Multiplying By A Proper Factor.
If λ < 1 / 4 then r1 and r2 are real and distinct, so the general solution of the differential equation in equation 13.2.2 is y = c1er1t + c2er2t. Where is a constant and is a known function called either the density or weighting function. The functions p(x), p′(x), q(x) and σ(x) are assumed to be continuous on (a, b) and p(x) >. If the interval $ ( a, b) $ is infinite or if $ q ( x) $ is not summable.
P, P′, Q And R Are Continuous On [A,B];
We can then multiply both sides of the equation with p, and find. We apply the boundary conditions a1y(a) + a2y ′ (a) = 0, b1y(b) + b2y ′ (b) = 0, There are a number of things covered including: However, we will not prove them all here.
Web The General Solution Of This Ode Is P V(X) =Ccos( X) +Dsin( X):
E − x x y ″ + e − x ( 1 − x) y ′ ⏟ = ( x e − x y ′) ′ + λ e − x y = 0, and then we get ( x e − x y ′) ′ + λ e − x y = 0. We will merely list some of the important facts and focus on a few of the properties. The boundary conditions require that The solutions (with appropriate boundary conditions) of are called eigenvalues and the corresponding eigenfunctions.
We Just Multiply By E − X :
Share cite follow answered may 17, 2019 at 23:12 wang (c 1,c 2) 6= (0 ,0) and (d 1,d 2) 6= (0 ,0); Α y ( a) + β y ’ ( a ) + γ y ( b ) + δ y ’ ( b) = 0 i = 1, 2. P and r are positive on [a,b].